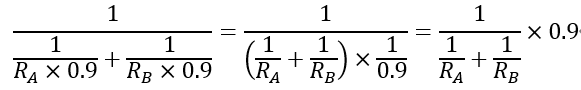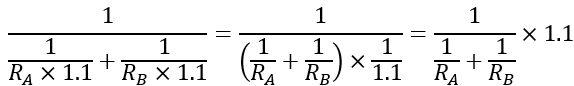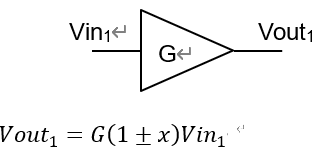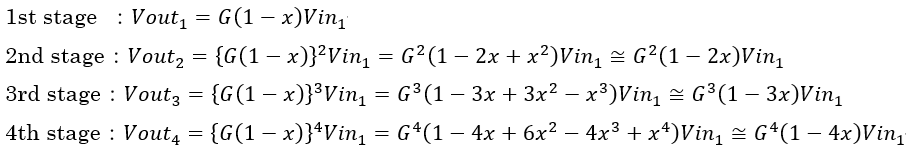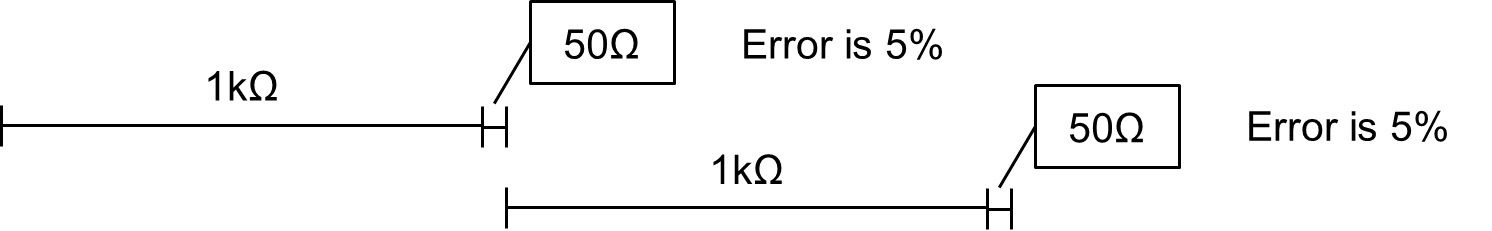[Correct answer]

（ｂ）　

 

[Explanation]

 This question is about the accuracy (tolerance) of a single resistor or a circuit made by combining several resistors.

 

●Consider the tolerance of series connection

 

As explained in the body of the question, when resistors with the same tolerance are connected in series, the tolerance of the combined resistance is the same as that of the original resistance. For example, when a resistor A with ±10% tolerance and nominal value of R_A and a resistor B with ±10% tolerance and nominal value of R_B are connected in series, the nominal value of the combined resistance is R_A+R_B and the tolerance is ±10%.

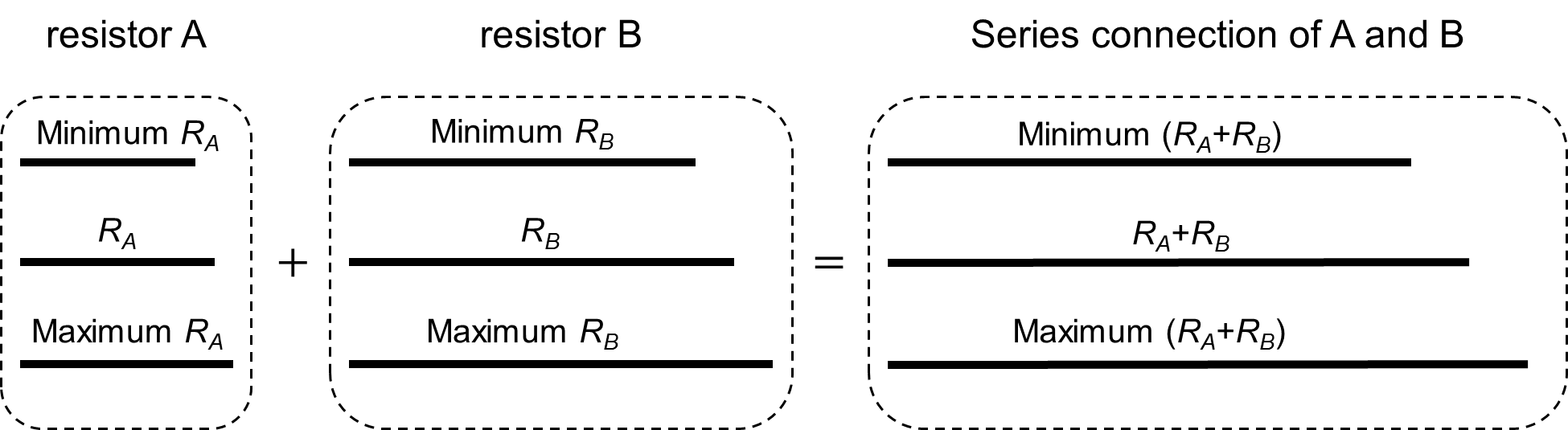
 Let us consider this as shown in Figure 1.

 Though the values of resistors A and B vary within their tolerances, the combined resistance is the sum of those values, so that A+B is also minimum when A is minimum and B is minimum. Similarly, A+B will be maximum when A is maximum and B is maximum.

 

Figure 1　Tolerance for series connection

 

Considering the formula, when the tolerance is ±10%, the minimum R is 0.9 times the nominal R, and the maximum R is 1.1 times the nominal R. Therefore, the following formula is used to calculate the minimum R and the maximum R.

　When R_A and R_B are minimum:

　　

　When R_A and R_B are maximum:

　　

 Then the minimum value of combined resistance is (R_A+R_B ) ×0.9 and the maximum value is (R_A+R_B ) × 1.1. That is, the minimum value is 0.9 times the nominal value of R_A+R_B and the maximum value is 1.1 times the nominal value of R_A+R_B. Therefore, the tolerance of the combined resistance is ±10%.

 The above results in the following

(1) When a resistor with ±10% tolerance is connected in series, the total tolerance is also ±10%.

 

●Consider the tolerance of parallel connection

 In the case of parallel connection, the combined resistance is in the form of a fraction, , as shown in Figure 2.

 It’s hard to understand as it is, so let’s first think about  on the denominator side.

Figure 2　Combined resistance of parallel connection

 

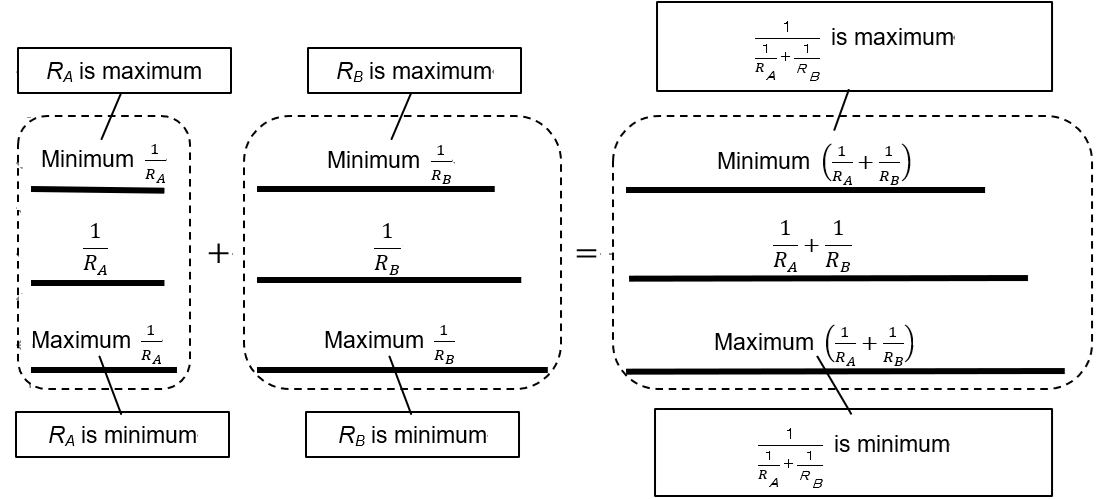
  As shown in Figure 3,  is minimum when  and  are both minimum, and  is maximum when  and  are both maximum.

 

 

Figure 3　Tolerance for parallel connection

 

 If we note that  is minimum when R is maximum and the inverse  is maximum when R is minimum, we can say that,  is maximum when R_A and R_B are both minimum and  is minimum when R_A and R_B are both maximum.

 Furthermore, since the combined resistance of the parallel connection is  , which is the reciprocal of , we can say the following.

(2) When R_A and R_B are both minimum, parallel connection is minimum.

(3) When R_A and R_B are both maximum, parallel connection  is maximum.

 

Next, let’s consider the calculation formula.

        When R_A and R_B are minimum：

        When R_A and R_B are maximum：

 Therefore, it can be seen that the minimum value of the combined resistance of parallel connection is 0.9 times the nominal value, and the maximum value of the combined resistance of parallel connection is 1.1 times the nominal value. As in the case of series connection, we can say the following.

(4) If resistors with a tolerance of ±10% are connected in parallel, the overall tolerance will also be ±10%.

 

●Considering option A

 Here, we will consider option A of the question statement.

 Option A says, “If two resistors with a tolerance of ±5% are connected in parallel, the combined resistance will be maximum when both are at nominal value -5%, and the combined resistance will be the minimum.when both are at nominal value +5%. “

 When the tolerance is ±5% and the two resistors are both at nominal value -5%, so from (2) the combined resistance will be the minimum. Therefore, the statement “The combined resistance will be maximum when both the nominal value -5%” is incorrect.

 When the tolerance is ±5% and the two resistors are both at nominal value +5%, so from (3) the combined resistance will be the maximum. Therefore, the statement “The combined resistance will be minimum when both the nominal value +5%” is incorrect.

 Therefore, option A of the question statement is incorrect.

 

●Considering option B

 Here, we will consider option B of the question statement.

 Option B says, “If you create a circuit by connecting multiple resistors with a tolerance of ±5% in series or in parallel, the error in the combined resistance of that circuit will fall within the range of ±5%.”

 Both (1) and (4) above are explained using the case of a tolerance of ±10% as an example, but they generally also hold true when the tolerance is ±x%. Therefore, whether two resistors with a tolerance of ±5% are connected in series or two resistors with a tolerance of ±5% are connected in parallel, the error in the combined resistance will be within ±5%.

 Furthermore, let’s consider a complex connection using three or more resistors.

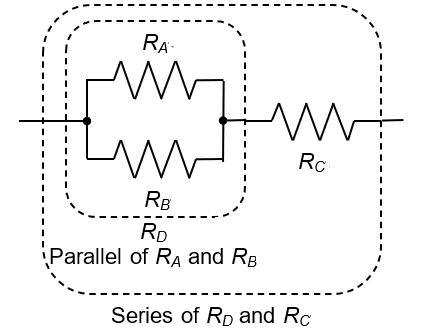
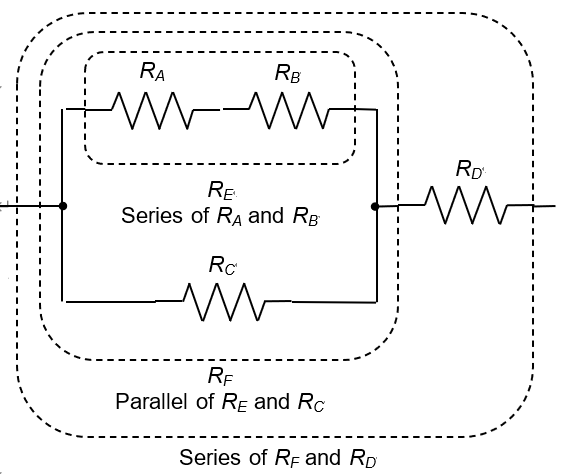
 For example, the circuit in Figure 4 that connects three resistors R_A, R_B and R_C can be thought of as first connecting R_A and R_B in parallel to form R_D, and then connecting R_C in series. Similarly, the circuit in Figure 5, which connects four resistors R_A, R_B, R_C and R_D, first connects R_A and R_B in series to form R_E, then connects R_C in parallel to form R_F, and then connects R_D in series. 

 Many circuits made by combining multiple resistors can be broken down into combinations of series connections and parallel connections like this. Since the original tolerance is maintained for each series connection and parallel connection, the original tolerance is also maintained for the whole of such a circuit. For example, a circuit made by connecting multiple resistors with a tolerance of ±5% in series or in parallel has a tolerance of ±5% as a whole, and the error of the combined resistance falls within the range of ±5%.

 Therefore, option B of the question statement is correct.

 

Figure 4　Example of series-parallel connection of 3 resistor

 

Figure 5　Example of series-parallel connection of 4 resistors

 

 Note that some circuits that use multiple resistors cannot be broken down into combinations of series connections and parallel connections. Even in that case, if the tolerance of each resistor is the same, the tolerance of the entire circuit can be considered to be the same as the tolerance of each resistor.

 

●When errors are accumulated, and when they are not

 It is often said that “errors accumulate in analog circuits.” This refers to the fact that, for example, when audio is repeatedly recorded and played back on an analog tape recorder, the signal deteriorates each time it is copied.

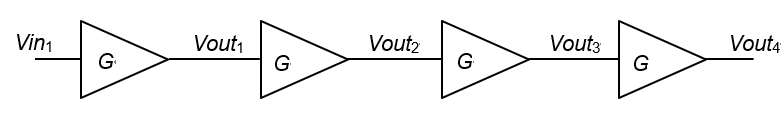
 As an example of a circuit, consider the case where an analog amplifier circuit with a gain error x as shown in Figure 6 is connected in series with N stages as shown in Figure 7. Here, we will consider the case where all errors in each stage are –x as the worst case, but it is the same even if all errors in each stage are +x.

 

Figure 6　Amplifier circuit with gain error

 

Figure 7　Example of series connection of amplifier circuits with gain error

 

 If the gain of the first stage is  and the gain of the second stage is also  , the combined gain of the first and second stages is  . Here, since x is an error, it is considered to be much smaller than 1 (for example, 0.1 or 0.01), so the term  is extremely small compared to  .

 Therefore, the combined gain of the first and second stages can be said to be approximately  .

 

 Similarly, the combined gain from the 1st stage to the 3rd stage is approximately  , and the combined gain from the 1st stage to the 4th stage is approximately  . You can see that the error x accumulates as it increases.

 ”Errors accumulate in analog circuits” refers to this situation.

 In contrast, series-parallel connection of resistors does not accumulate errors. For example, if you connect resistors in series with a nominal value of 1kΩ and an error of +50Ω (+5% for 1kΩ) as shown in Figure 8, the errors will add up to +100Ω, but the nominal value will also be added. Since they add up to 2kΩ, the ratio between the nominal value and the error remains unchanged.

 

 

Figure 8　In series connection, both nominal value and error are added

 

●Considering option C

 Here, we will consider option C of the question statement.

 Option C says, “If you connect a number of resistors with a nominal value of 1kΩ and a tolerance of ±5% in series, the error in the combined resistance will become larger than ±5% due to the accumulation of errors. “

 As we have seen so far, when resistors with the same tolerance are connected in series, the tolerance of the combined resistor is the same as the tolerance of the original resistor. If N resistors of 1kΩ, ±5% are connected in series, the nominal value of the combined resistance will be NkΩ, and the tolerance will remain ±5%.

 Therefore, option C in the question statement is incorrect.

 

●Consider value variations and tolerances

 As explained in the body of the question, the actual resistance value of the resistor has some error, and it is not exactly the nominal value, but has variations. The range of variation from the minimum to the maximum is the tolerance. Therefore, for example, if a resistor has a nominal value of 1kΩ and a tolerance of ±1%, the actual resistance value could be any value between 990Ω (1kΩ-1%) and 1010Ω (1kΩ+1%).

 This variation results from physical variations during the resistor manufacturing process. Generally, as shown in Figure 9, the frequency is higher near the center of the range, and the frequency is lower near ends of the range. Among these distributions, the most well-known form is the normal distribution. Although actual resistors do not have a normal distribution, they are expected to have a similar distribution.

Figure 9　Example of resistance value distribution of an actual resistor

 

 Here, when comparing the distributions of tolerance ±1% and tolerance ±5%, it can be seen that ±1% is often closer to the nominal value, and ±5% is often farther from the nominal value. However, there are values that are extremely close to the nominal value even at ±5%, and there are values that are far from the nominal value at ±1%. In other words, a ±1% resistor is not always closer to its nominal value than a ±5% resistor.

 

●Considering option D

 Here, we will consider option D of the question statement.

 Option D says, “If you compare the actual values of a resistance with a nominal value of 1 kΩ and a tolerance of ±1% and a resistance with a tolerance of ±5%, the ±1% resistance is always closer to 1 kΩ. “

 As explained above, there are resistors with a ±5% value that are extremely close to 1kΩ, and there are resistors with a ±1% value that are further away from 1kΩ. A ±1% resistor is not always closer to 1kΩ.

 Therefore, option D in the question statement is incorrect.

 

 To summarize the above, among the options, A, C, and D are incorrect, and B is the correct. The correct answer to this question is B